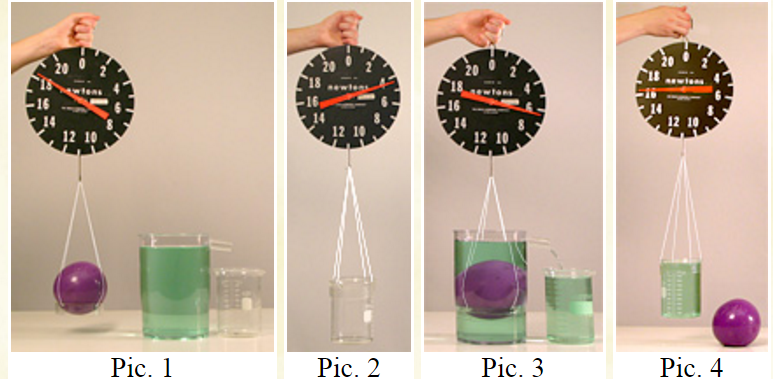
Materials:

• Newton scale
• Plastic ring attached to strings to weigh beaker with
• 1 L glass beaker
• Heavy purple ball
• Hollow rubber ball

Part 1

Procedure:

1. Place the heavy ball onto the plastic ring and weigh it on the Newton scale. Make sure the students can see the result of the measurement. (Pic. 1)
2. Weigh the empty glass together with the plastic ring. (Pic. 2)
   Place the heavy ball onto the ring suspended from the scale, then immerse it into water.
3. Wait until all water displaced by the ball pours into the glass. Show the students the weight loss of the ball while it is submerged in water. (Pic. 3)
4. Weigh the glass filled with displaced water. (Pic. 4)
5. Calculate the weight of displaced water by finding the difference between weights of an empty and a filled glass.
6. Show students that the weight of the displaced water equals the weight lost by the submerged heavy ball.

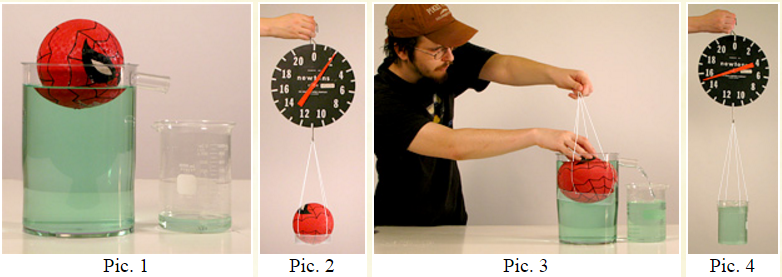
Part 2
Procedure:

1. Place the hollow rubber ball in the water to demonstrate that it floats. Point out that it displaces only a small amount of water. (Pic. 1)
2. Place the rubber ball onto the plastic ring and weigh it on the Newton scale. (Pic. 2)
3. Weigh the small amount of displaced water and show that the weight of the ball is equal to the weight of the displaced water.
4. Submerge the rubber ball by forcing it down into the water with your hand. (Pic. 3)
5. Wait until all water displaced by the ball pours into the glass.
6. Weigh the filled glass and calculate the weight of displaced water. For the weight of the empty glass use measurement obtained in part 1, step 2. (Pic. 4)
7. Show the students that the weight of displaced water when the ball is forced under the water is much greater than the weight of the rubber ball.

Explanation:

This demonstration exemplifies Archimedes’ principle: the upward buoyant force that is exerted on a body immersed in a fluid, whether fully or partially submerged, is equal to the weight of the fluid that the body displaces. This buoyant force acts in the upward direction at the center of mass of the displaced fluid.

First we should define the buoyant force. The buoyant force (often referred to as simply buoyancy) is the upward force exerted by a fluid that opposes the gravitational force on an immersed object. While the buoyant force is exerted on all objects, irrespective of their weight or density, it is much easier to feel when the object that is displacing the fluid has a very low density (a balloon as opposed to a bowling ball, for example). You’ve felt the buoyant force if you’ve ever floated on an inner tube or tried to push an air-filled ball into a tub of water. The harder you attempt to submerge the object, the greater force you feel in response. This is because as you displace more water, the buoyant force will be greater.

This is because fluids are not a consistent density all the way through; there is an increase in the density the farther down into the fluid you go. The weight of the overlaying fluid causes the pressure at the bottom of a column of water to be significantly greater than the pressure at the top of the column. This effect is the same as that which causes you to feel the pressure difference in an airplane. Air is a comparatively low density fluid, however, so you need to travel a large vertical difference to feel the pressure change. With a dense fluid like water, the pressure change is noticeable with a fairly small Δy.

The difference in pressures within the fluid causes an object to feel a pressure gradient when submerged in the fluid (i.e., there is greater pressure at the bottom of an object immersed in a fluid than at the top). This pressure difference is what results in the upward force on the object. When an object is shaped appropriately, the upwards force can cause it to float (like a small boat of lead foil, which is ordinarily much more dense than water).

For floating objects that are not entirely submerged, the weight of the displaced fluid is equal to the weight of the object itself. However, for an object that is completely submerged (and whose density is not exactly equal to the density of the fluid), the volume of the fluid displaced is equal to the volume of the object displacing the fluid.This law can be used to find the volume of irregularly shaped objects by finding the weight of the displaced fluid and then using the density of the fluid to find the volume displaced.

In the first part of the demonstration we show that a ball that is completely submerged in water weighs less on the newton scale than it did when it was simply in air. This is because the newton meter will measure the net force pulling down on it. When in air, this is equal to the mass of the object times the acceleration of gravity (F = ma); when in water, on the other hand, you also have the additional buoyant force that partially counteracts the gravitational force on the ball.

Written by Sophia Sholtz